how to find the domain of a graph
Find the domain of a graph of a function; examples with solutions are presented. First the graphical meaning of the concept of the domain of a function is explained.
Domain of a Graph of a Function
The implied domain of a function f is the set of all values of x for which f(x) is defined and real. The graph of a function f is the set of all points (x , f(x)). Hence, For a function f defined by its graph, the implied domain of f is the set of all the real values x along the x-axis for which there is a point on the given graph.As an example there are points on the graph below at x = - 3, - 2.5, -2, -0.5 , 2,5, 3, 3.2, 4. These values and more other values of x are included in the domain of f.
There are no points on the graph at x = - 1 (open circle on the graph) , 0.5 , 1, 1.5 , 2 (open circle). These values and more other values of x are not included in the domain.



With these ideas and definition, we will now solve examples where the whole domain of a given graph is found.
Domain of a Graph; Examples with Detailed Solutions
Example 1
Find the domain of the graph of the function shown below and write it in both interval and inequality notations.
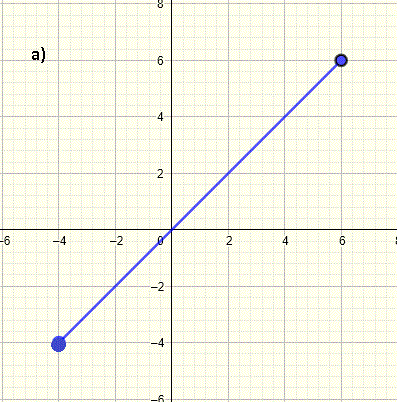


The graph starts at x = - 4 and ends x = 6. For all x between -4 and 6, there points on the graph. Hence the domain, in interval notation, is written as
[-4 , 6]
In inequality notation, the domain is written as
- 4 ≤ x ≤ 6
Note that we close the brackets of the interval because -4 and 6 are included in the domain which is indicated by the closed circles at x = - 4 and x = 6.
Example 2
What is the domain, in interval notation, of the graph of the function shown below?
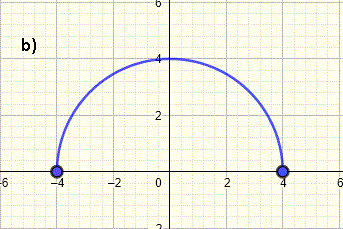


The graph starts at x = - 4 and ends x = 4. There are points on the graph for all values of x between - 4 and 4 including at - 4 and 4. Hence the domain, in interval notation, is written as
[ - 4 , 4]
Example 3
What is the domain of the graph of the function?
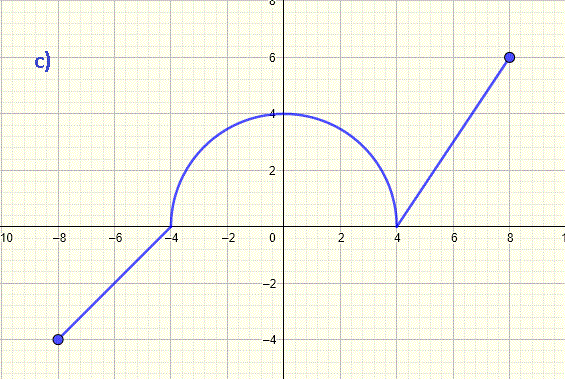


The graph starts at x = - 8 and ends x = 8. The graph is defined for all x between - 8 and 8. We include - 8 and 8 because of the closed circles at x = - 8 and x = 8. Hence the domain, in interval notation, is written as
[ - 8 , 8]
Example 4
Find the domain of the graph of the function shown below.
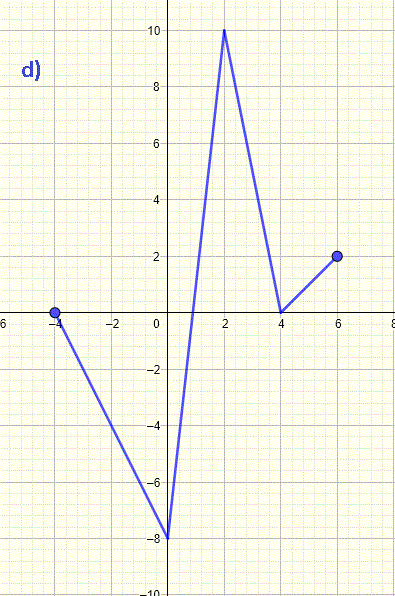


The graph starts at x = - 4 and ends x = 6. The graph is defined for all x between - 4 and 6. The interval is closed at - 4 and 6 because of the closed circles at x = - 4 and x = 6. Hence the domain, in interval notation is written as
[- 4 , 6]
Example 5
Write the domain of the graph of the function shown below in interval and inequality notations.



The graph starts at values of x > - 4 and ends at values of x < 4. x = - 4 and x = 4 are not included in the domain because of the open circles at these values. Hence the domain, in interval notation, is written as
( - 4 , 6 )
Note that the interval is open to indicate that - 4 and 4 are not included in the domain of the graph.
In inequality notation, the same domain is given by
- 4 < x < 6
Note that the strict inequality sign (without equal) is used in the inequality notation of the domain because x = - 4 and x = 6 are not included in the domain.
Example 6
Write the domain of the graph of the function shown below in interval notation.
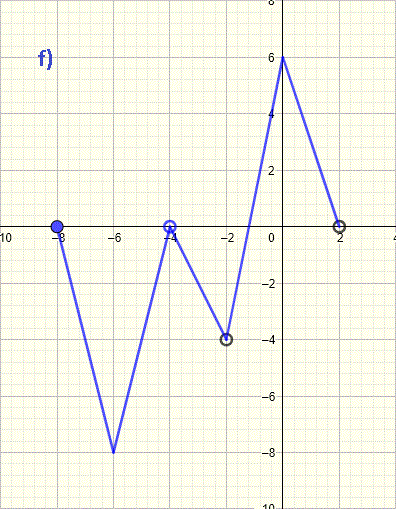


The graph starts at values of x = - 8 and ends at values of x < 2. The open circles at x = - 4, x = -2 and x = 2 indicates that these values are not included in the domain. Hence the domain, in interval notation, is written as
[ - 8 , - 4 ) ∪ (- 4 , -2) ∪ (-2 , 2)
Note the interval is open at x = - 4, x = -2 and x = 2 to indicate that these values are not included in the domain of the graph.
Example 7
Write the domain of the graph of the function shown below in inequality notation.
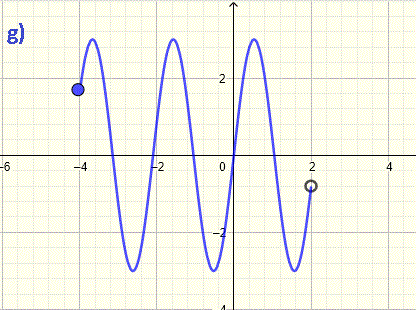


The graph starts at x = - 4 and ends x < 2. The domain does not include x = 2 because of the open circle at x = 2. Hence the domain, in inequality notation, is written as
- 4 ≤ x < 2
Example 8
Write the domain of the graph of the function shown below using interval notation
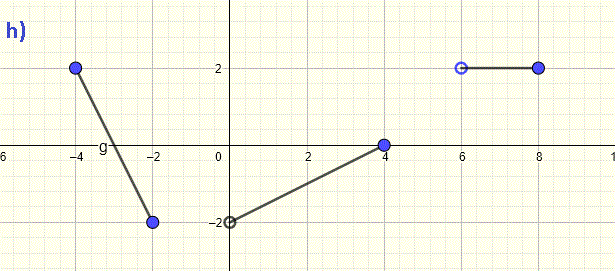


Solution to Example 8
The graph is made up of three parts. The left part is defined for all values of x between - 4 and - 2. The part in the center is defined on the interval x > 0 and x ≤ 4. The part on the right is defined for x > 6 and x ≤ 6. The domain is written as a union of three intervals as follows
[ - 4 , -2 ] ∪ (0 , 4] ∪ (6 , 8]
More Links and References
Find domain and range of functions,Find the domain of a function ,
Step by Step Solver to Find the Domain of the Square Root of a Linear Function,
Find the Domain of the Square Root of a Quadratic Function
how to find the domain of a graph
Source: https://www.analyzemath.com/DomainRange/domain-of-a-graph.html
Posted by: brunsmurdelize.blogspot.com

0 Response to "how to find the domain of a graph"
Post a Comment